
In the cryptocurrency options market, the bid-ask spread is an important factor that requires additional attention.
Usually, the most actively traded options are those expiring in 30 days with a bid-ask spread around 0.0005 BTC to 0.0015 BTC. For a better comparison, if the spread is divided by the premium, for at the money (ATM) options it is about 10% of the premium, while for out of the money (OTM) options, it can be as high as 20%.
If a trader opens and closes his option position by taking bid price and ask price, the total bid-ask spread he pays can accumulate to up to 20-40% of the premium. If he keeps on doing this frequently, his capital can get drained out fast.
There are two ways to reduce the cost of bid-ask spreads. One is to “hold until expiration”, thus closing the position with the minimum cost upon delivery. Or using limit orders when opening or closing a position, instead of taking market bids and offers. Nonetheless, while saving bid-ask spreads, the trader might miss out on some major trading opportunities.
In short, the relatively high bid-ask spreads of Bitcoin options can restrict traders from moving positions frequently, which can constrain efficient trading.
Hold Until Expiration
Let’s use a recent Bitcoin chart as a case study. Bitcoin price was at $9,600 at around 23:00 UTC on May 28, 2020. The price remained at this level for about 4 days – until June 1, 2020.
If “Trader A” at that time had bought a weekly OTM call option, with the strike price at $9,750 and the expiry date June 5, he would have suffered a stable loss on Theta in the past four days (Theta is the Greek letter used for daily option time value).
However, after some long and painful waiting, the Bitcoin price finally skyrocketed at the midnight of June 1. Within 3.5 hours from 20:00 to 23:30, it surged from $9,600 to $10,400. The premium of call options could have tripled or increased even more.
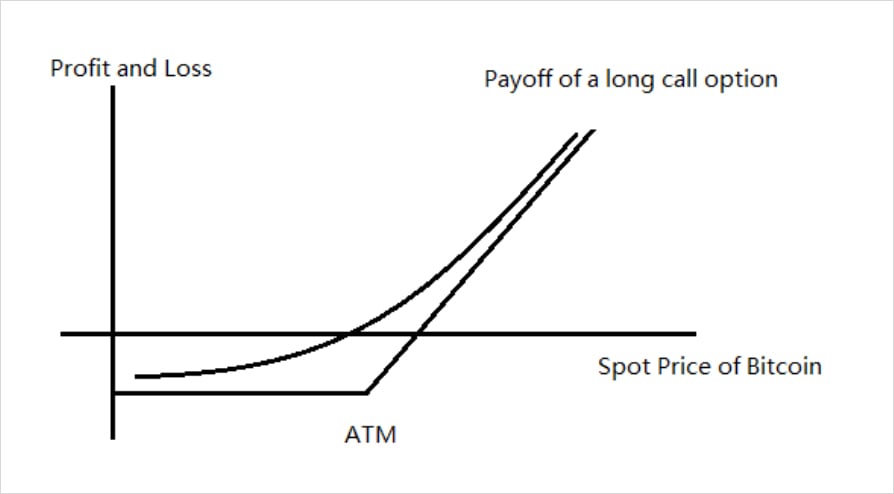
Convexity of a Call option: The more underlying moves upwards, the faster profits accumulate.
By that time, trader’s Call option was already in the money (ITM) and the ITM option’s premium was even higher, as it now had intrinsic value.
The intrinsic value is the value where the price of the underlying exceeds the strike, which then requires an extra margin to trade it, and especially to sell it. This is the reason why ITM options are always less liquid and the bid-ask spread can be too large to trade. It could be 0.0050 BTC or even more. For deep ITM options, there might not even be a price to take on the quotation screen.
Due to this, “Trader A” decided not to close the position outright and to hold it till expiration. After all, it’s a weekly option and it would get settled soon. However, after half a day, BTC price fell back to $9,600, and on June 2, all the profits of the call option were gone.
Let’s suppose Trader A continued holding. At 08:00 June 5, when the options got exercised the Bitcoin price was $9,800, and Trader A made $50. Considering the premium paid upon buying the Call (about $200), this position lost money in the end.
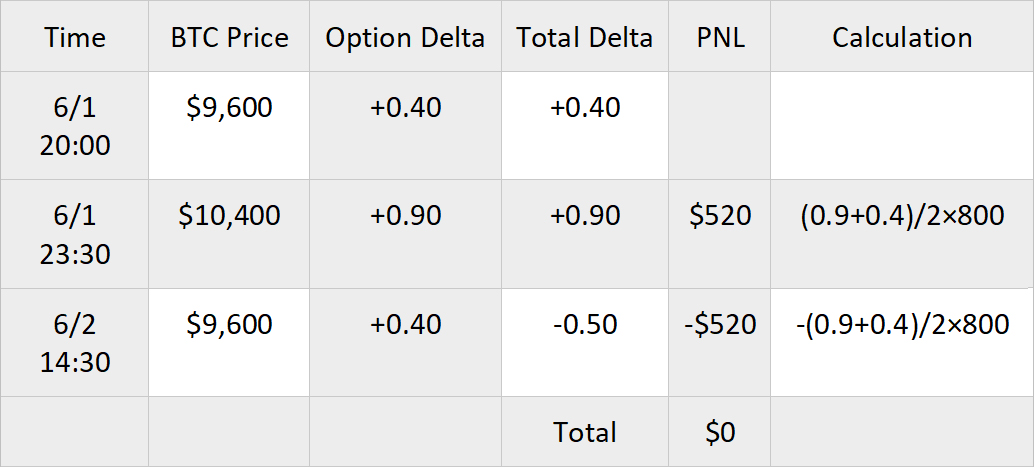
Table 1: PNL (Profit and Loss) of Trader A
Hedging Delta to Lock Profit
If the trader had adjusted the directional exposure (Delta) to zero after every big change of the underlying asset’s price, by buying or selling the corresponding number of perpetual contracts or futures contracts, this story would have had a different ending.
Suppose that in the process of BTC price going from $9,600 to $10,400, “Trader B” had only one call option position, and the Delta of the Call option gradually changed from +0.40 to +0.90. This meant that the directional PnL of the option was equivalent to going long 0.40 to 0.90 perpetual or futures contract. In this process, if we use the arithmetic average to estimate the average Delta, it would have been be (0.4+0.9)/2 = 0.65.
This calculation is a rough estimation but can give an intuitive idea.
If we multiply the directional exposure, i.e. Delta, by the distance of the underlying asset’s (i.e. Bitcoin) price move, we can estimate the profit during this drift: 0.65 * $800 = $520.
Till this point, the profit and loss of Trader A and Trader B would have been the same.
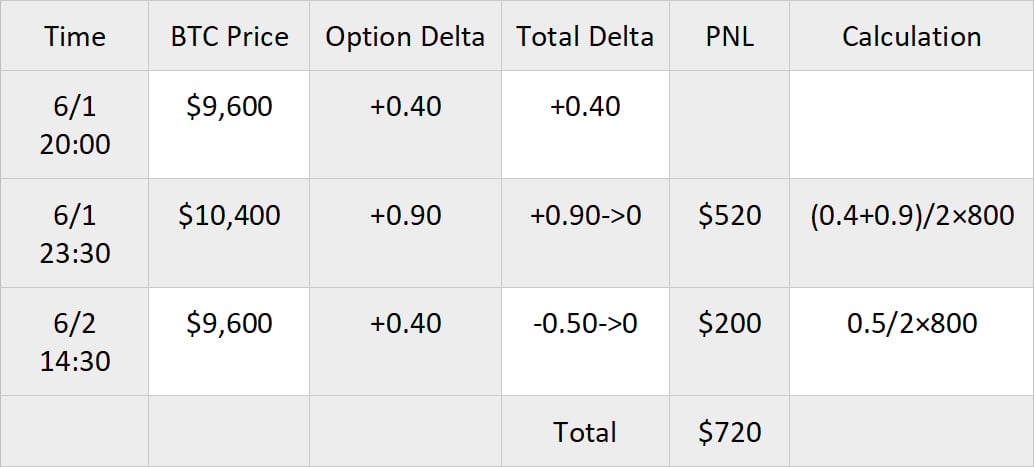
Table 2: PnL of Trader B
However, then Trader B did something different. At $10,400, the directional exposure or Delta of the option was +0.90. Then Trader B believing that the massive price drift was over, sold 0.90 BTC perpetual or futures so that the directional exposure of the portfolio would become zero again.
Apart from option Delta, option premium settled in BTC would also have an impact on the portfolio’s PNL. However, for simplicity, we will neglect this impact for now and we may analyze this phenomenon in future articles.
Because of convexity (or “Gamma”), Delta will become larger as BTC price rises and smaller as BTC price falls. So what should we do in the face of a changing Delta?

Delta of a Call option and Convexity
Let’s Analyze It Step By Step
Scenario 1, BTC price rises. Delta of the option changes from +0.90 to +1.00, while futures Delta remains at -0.90. Total Delta of the portfolio changes from 0.00 to +0.10. BTC price rises, Delta is positive, and the portfolio is making money.
Scenario 2, BTC price falls. Delta of the option changes from +0.90 to +0.80, while the futures Delta remains at -0.90. Total Delta of the portfolio changes from 0.00 to -0.10, a negative number. BTC price falls, Delta is negative, and the portfolio is still making money.
In either scenario, the portfolio is profitable. If a trader neutralizes the portfolio’s delta each time there is considerable delta exposure, the profit is locked up and new profit will be made no matter what direction the underlying asset takes next.
Now let’s look back to our case study of Trader A and Trader B when BTC price fell from $10,400 to $9,600, Trader A’s profit had sadly returned to zero, not even mentioning the Theta cost.
On the other hand, the total Delta of Trader B gradually changed from 0 to -0.50. Why was that? As the option’s Delta decreased from +0.90 to +0.40, the future’s Delta remained unchanged at -0.90. So altogether it was +0.40-0.90 = -0.50.
So what did the Trader B make during this process? The average Delta multiplied by the drifted distance here is 0.50/2 * $800 = $200.
While the market went up and down. Trader B earned $520 + $200 = $720, however, Trader A earned nothing and even lost some.
Starting From Neutralized Delta
The example given above may seem deliberate. Trader B might have taken the wrong direction in the beginning, in which case instead of earning the first $520 profit, he could have potentially suffered a loss.
Therefore, let’s see what Trader C could have done differently. He could have adjusted Delta to neutral at the initial point and rebalanced Delta after each significant price drift.
At $9,600, Trader C could have shorted 0.40 perpetual or futures, causing the portfolio’s Delta to drop to zero.
At $10,400, Call Delta would have gone from +0.40 to +0.90, while the future’s Delta would have remained at -0.40. Thus, making total Delta +0.50. In this process, Trader C would have made $200. Then he could have further shorted 0.50 perpetual or futures contract, causing the future’s Delta to drop to -0.90, and making portfolio’s total Delta neutral again.
On the way back to $9,600, Call Delta went from +0.90 to +0.40, whereas the futures Delta remained at -0.90. In this case, the total Delta was -0.50. Due to this, Trader C would have made another $200.
With the underlying asset’s price going back and forth, a profit of $400 was made. It is not as high as Trader B’s though, as Trader C gained less due to his lower risk exposure at the starting point.

Table 3: PnL of Trader C
Summary
Option buyers can continuously accumulate profits by starting with a neutralized total Delta and dynamically hedge portfolio’s Delta whenever there is a major price drift. This trading strategy is called “Gamma Scalping”. According to experience, this strategy can recover about half of the premium if the market stays quiet, effectively making option buyers more robust, and increasing their chance of holding on and making a big hit when a major market move finally comes. The liquidity of Bitcoin perpetual or futures contracts is significantly better than that of options. By applying dynamic delta hedging (DDH), traders can control and minimize the problems coming from high bid-ask spread of options trading.
How About Automated Delta Hedging?
If you would like to simplify Your trading, you can use Greeks.Live App. It is a trading app integrated with Deribit that provides a convenient feature called “delta-hedging with one click”. With one click traders can easily enter the hedge transaction confirmation interface, manually trigger a hedge transaction, and make the Delta neutral. This can help to avoid the trouble of inputting corresponding contract size and fat finger situation.
AUTHOR(S)
